“Where do we start, from the basics the numbers, maths” “Maths Oh no, it is the subject which invokes a strong reaction from people -one either hates it or loves it one cannot be neutral to it.” Yes though people hate maths and there is more to maths than numbers . We need to go through some basic concepts. Are you in?
“Yes I am”, replied Swayam.
“Are you ready?”, asked the voice. “We know about the numbers 0-9 and the face value and the base value and basic mathematical operations -addition, subtraction, multiplication and division”.
“Right,” said Swayam.
“The most widely used concept of mathematics that is used in the financial world is Percentage or percent. Percentages are used in our everyday life and we may not even realize it !!”, said the voice.
Table of Contents
Percentage
In sales, for example a shop is having a 20% off sale.
Or
You deposit 5000 in a bank you get a 8% interest.
Percentage is a way of expressing a number as a fraction of 100. It is often denoted using the percent sign, “%”.
For example, 45% is read as “forty-five percent” and is equal to 45/100, or 0.45.
Swayam getting worked up “Hey I know all this. I was taught all this in school. Let’s find 30 percent of 400: 30% = 0.30 so 0.30 x 400 = 120”
“Sorry” said the voice , “so you can do the following right. In a department store, a dress is marked for Rs 800 with discount of 25%.” What is the discount? What is the sale price of the dress? “Yes, simple”, said Swayam ,”25% of 800 is Rs 200 so discount is Rs 200 and dress would cost Rs 600. Don’t tell me kiddish stuff.”
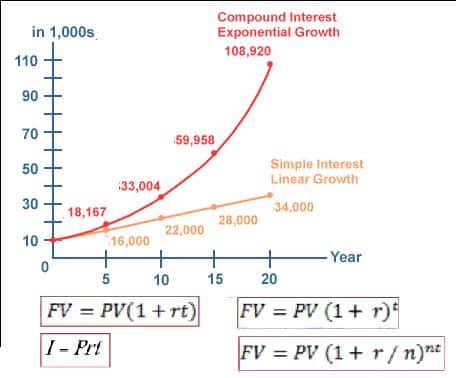
Simple Interest and Compound Interest

- Simple Interest and Compound Interest Comparison
Rs 100 a day or Rs 1 on first day doubling the next day
Voice asked, “Rs 100 a day or Rs 1 on first day doubling the next day, I mean Rs 2 on second day, Rs 4 on third.” Swayam asked “What kind of question is that? I am sure there is some trick to it?” “You are right,” voice said, “Let me tell you a story.”
Once upon a time, a king lost a game of chess to a farmer. The king asked the farmer to choose his reward and all that the farmer asked for was one grain of wheat for the first square of the chess board, two grains for the second square, four grains for the third, eight for the fourth and so on and so forth for all the 64 squares. The king was very happy for being let off rather lightly and readily granted the wish. To see the how many grains of wheat are in each square see the picture below:
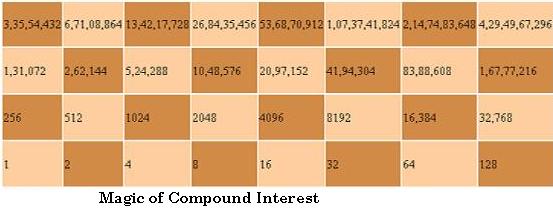
“Wow that is interesting. Now I understand why Albert Einstein, the great scientist, called Compound Interest Eighth wonder of the world”.
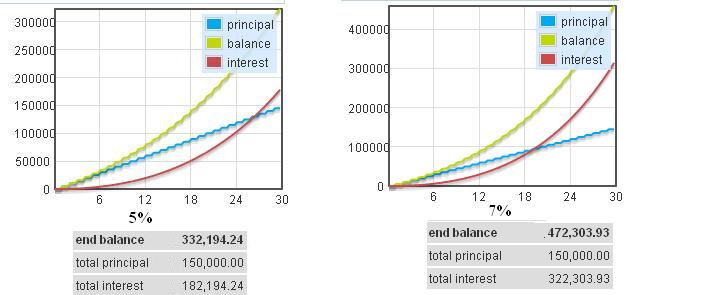
Periodic Compounding
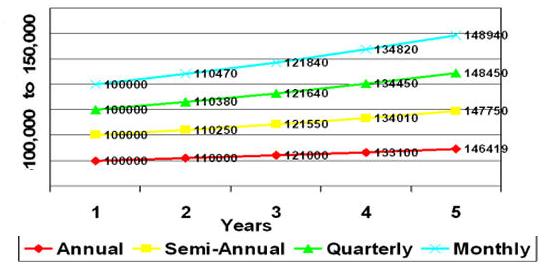
As you can tell from the above graph, the 100,000 that is compounded monthly grows much faster than the 100,000 that is compounded annually. In a matter of 5 years: 100,000 -> 148,940 (Compounded Monthly) and 100,000 -> 146,419 (Compounded Annually). Difference = 148,940 – 146,419 = 2521!
2% Difference
Rule of 72
“Hey I cannot do these calculations fast and this is putting me off”, grumbled Swayam. ” I know but there is a shortcut,” replied the voice. “Called Rule of 72”. “Wow..tell me more about it,” said Swayam. The voice said, “Rule of 72 is a shortcut formula to find out approximately in how many years amount will double? Divide 72 by rate of return you will get the number of years in which your money will double. For example, if you want to know how long it will take to double your money at eight percent interest, divide 8 into 72 and get 9 years. If you expect a rate of return of 12% you money will double in 6 years (72/12=6).
This can also be used in reverse order at what rate your money will double in 5 years – 72/5=14.4%
Rule of 114 will tell approximately in how many years your money will be triple & Rule of 144 approximately in how many years your money will quadruple.
Try the calculators at:http://www.moneychimp.com/features/rule72.htm
“Wow,” said Swayam, “that was good. I want to learn more..” Thanks Swayam. The next lesson is about it’s not what you earn but what you save..Remember An uneducated individual armed with a credit card and access to a mortgage can be just as dangerous to themselves and their community as a person with no training who is given a car to drive.”
You might be interested to read about :
- Educated but have No Financial Education
- KBC: Who pays for prize money and more
- What my car taught me about Personal Finance
- Three stages of investment and Tax
- Saving Bank Account:Do you know how interest is calculated and more
Did you like this article? Did it clarify your doubts about percentages, interest, simple interest, compound interest? Did it lack something? What part of finanical investment confuses you?




extensively written article
i remember reading Rich Dad Poor Dad & thinking this makes a lot of sense. Our education hardly touches upon financial intelligence which is actually more critical
Thanks Sujata. Yes I am big fan of Rich Dad Poor Dad. Wish we knew basics of finance in school!!